How to Solve Problems by Starting with the End and Working Backward

If you were to teach a youngster the game of chess, how would you go about doing it?

If you’re like most chess tutors and coaches, you’d start with the opening, then move to the middle part of the game, and then finally teach the endgame. It makes sense, right? The game starts with the openings and then moves forward linearly, and so it would only be fitting to follow that pattern in how you teach it. Similarly, if you’re teaching history, you begin by teaching the ancients; if you’re teaching writing, you begin by teaching how to write the first line.

But that’s not how the best minds in the game approach it. If you begin by teaching the openings, it won’t click. The inquisitive student will wonder why certain openings are the way they are and what makes them better or worse. You’ll quickly find that this is a practically limitless exercise. The game’s opening set-up is fairly simple with only ten possible moves for each player. But, by the time each player has made just four moves, there are more than 318 billion possible combinations. It is said that the number of potential complete chess games exceeds the number of particles in the known universe.

As you might guess, the infinite possibilities can be overwhelming, especially for a new player. How do you know what opening to use if you don’t know what middlegame you’re trying to get to? And how do you know what middlegame to use if you don’t know what endgame you prefer? The fact is, you cannot answer those questions without first examining the ultimate goal of the game—the checkmate. 

Thinking from the End

The best way to frame the problem, then, is to start with the end and work backward. Start by teaching the checkmate and what are called ‘mating patterns’, a set of a dozen or so key moves you can use to win the game. Then, using the checkmate as your reference point, you can figure out what position allows you to achieve those checkmates. Once you know what endgame set-up to aim for, then you know how to approach your middlegame. And, once you know what middlegame to aim for, you can use that to know what approach to use in your opening.

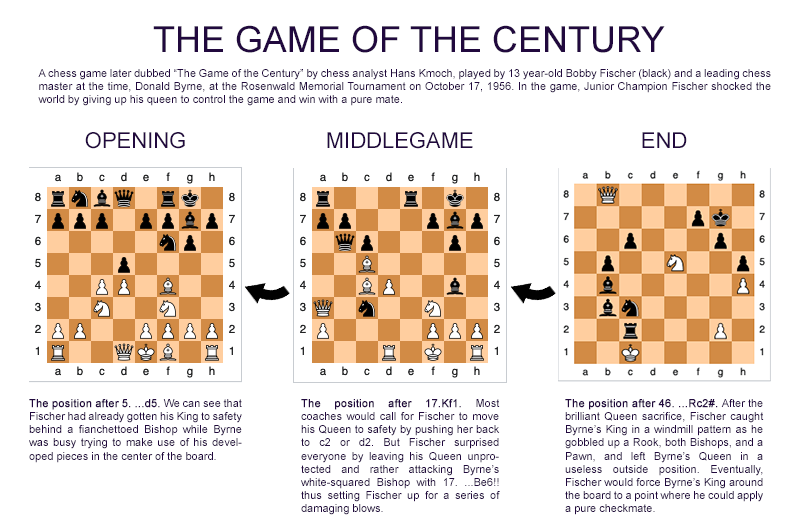
There are many theories as to why players like Bobby Fischer, Gerry Kasparov, and Magnus Carlsen are so good at chess, but one essential ingredient is this: They view the game from the perspective of the ultimate goal—the checkmate—and build their strategy around it from the very opening. Top players have mastered what positions will provide the best opportunity for checkmate and so marshal all their forces toward achieving those positions.  

And so, if you are to coach a youngster to be a grandmaster, it makes sense to teach it from their perspective, what GM Melikset Khachiyan calls “thinking from the end”. This is why the first and longest chapter in Fischer’s book is titled ‘Elements of Checkmate’. And it is why the Russian school of chess, long considered the premier program in the world, starts by coaching the endgame. Only when you know your endgame can you make sense of the infinite possibilities before you at the start of the game.

An Inverted Approach

Starting with the end and working backward is counter-intuitive because we are linear thinkers. But chances are you’ve used it in your own day-to-day life. One common example is preparing for an event. If you have to catch a flight at 2 p.m. for instance, you will start with a 2 p.m. departure as your goal and work backward from there to know when you need to start getting ready. You know you want to be at the gate at latest a half hour before departure. You know that it takes at least 30 minutes to get through security, so you need to be at the airport by 1 p.m. You have a rental car to return, which you figure will take 30 minutes all told, and you find that traffic is heavy midday, so it will take 45 minutes to drive to the airport. That means that you have to be out of the house by 11:45 a.m. Unless, of course, you’re my mom and leave the house at 7:30 a.m. just to be on the safe side.

The approach can also help with brain teasers such as the card trading game. Say there are six cards labeled ‘1’ through ‘6’ laid face-down before you. You and a partner each pick up a card. The goal is to obtain the highest number and are given the option to trade with your partner. Let’s say that you have picked up the ‘2’ card. Do you trade?

If you are thinking from the beginning, you might want to go ahead and trade—‘2’ is very low and the chances are that your partner picked up a higher card. But, if we think from the end, we gain a different perspective. If your partner wants to trade, it means he certainly does not have the ‘6’ card—the highest card—as doing so would mean giving you the win. Similarly, if he has the ‘5’ card, he’s going to hold on to that because he knows that you’re not going to trade if you have the ‘6’. By the same token, if ‘5’ and ‘6’ don’t trade, then he’s not going to trade if he has a ‘4’ either. Likewise, a ‘3’ is not going to trade because ‘4’, ‘5’, and ‘6’ don’t trade. And so, the offer must be a ‘1’ and you should definitely not trade.

As most people naturally work forward when trying to solve problems, working backward can be a powerful tool when you’re trying to problem solve in a competitive situation such as a game or business. In college, I won a stock market game using this very technique. The game was relatively simple: We could buy and sell different stocks with various volatility or we could purchase bonds that were stable. Each morning we would find out how our stocks did and could trade for other stocks or cash in for bonds. The stocks did really well, but there was a catch—it took a full day to sell the stocks for cash whereas we could cash in the bonds instantaneously. Working backward, I figured I would need all bonds the last day of trading. So, while everyone else was grabbing the high-flying stocks for the last trade, I converted everything to bonds and ended up the only one with cash at the end of the game. 

Conclusion

By working backward, we are able to gain perspective on the problem and hone in on the direction we want to take from the start. You’ll be amazed at how useful and versatile the approach can be. It works in puzzles as much as it does in getting to the airport. And, as we see, it works for the best chess players in the world.